Studying about shapes is a vital half of a kid’s early schooling. Whereas most youngsters are conversant in flat, two-dimensional shapes, introducing them to three-dimensional or stable shapes is an thrilling journey into the world of geometry. This information will take you thru the assorted varieties of stable shapes for youths and supply some attention-grabbing examples to make the educational course of enjoyable.
What Are Strong Shapes?
Strong shapes, sometimes called “stable shapes in maths,” are three-dimensional objects which have size, breadth, and top. Not like two-dimensional shapes, that are flat and have solely size and width, stable shapes have depth as nicely. They occupy house in the true world and may be touched, held, and moved round.
Youngsters encounter many of those shapes of their every day lives. As an illustration, a soccer ball resembles a sphere, whereas a cereal field may be likened to an oblong prism. The research of stable shapes in maths not solely enhances spatial consciousness but in addition builds the inspiration for extra superior geometric ideas.
To assist youngsters grasp the concept, listed below are some frequent examples of stable shapes:
1. Sphere: Consider planets or basketballs.
2. Cuboid (Rectangular Prism): Like bins or bricks.
3. Dice: The cube utilized in board video games is an ideal instance.
4. Cylinder: Tender drink cans and sure varieties of batteries.
5. Cone: Ice cream cones or visitors cones.
6. Pyramid: The traditional pyramids of Egypt or sure board recreation items.
Understanding these primary stable shapes lays the groundwork for youths to delve deeper into the world of geometry and to understand the myriad types and constructions they encounter of their environment.
Completely different Sorts Of Strong Shapes
Strong shapes are three-dimensional figures that occupy house. They’re throughout us, from the buildings we stay in to the toys children play with. Whereas there are quite a few stable shapes, we’ll be specializing in seven major varieties. Every form has its distinctive set of properties that defines it.
1. Dice
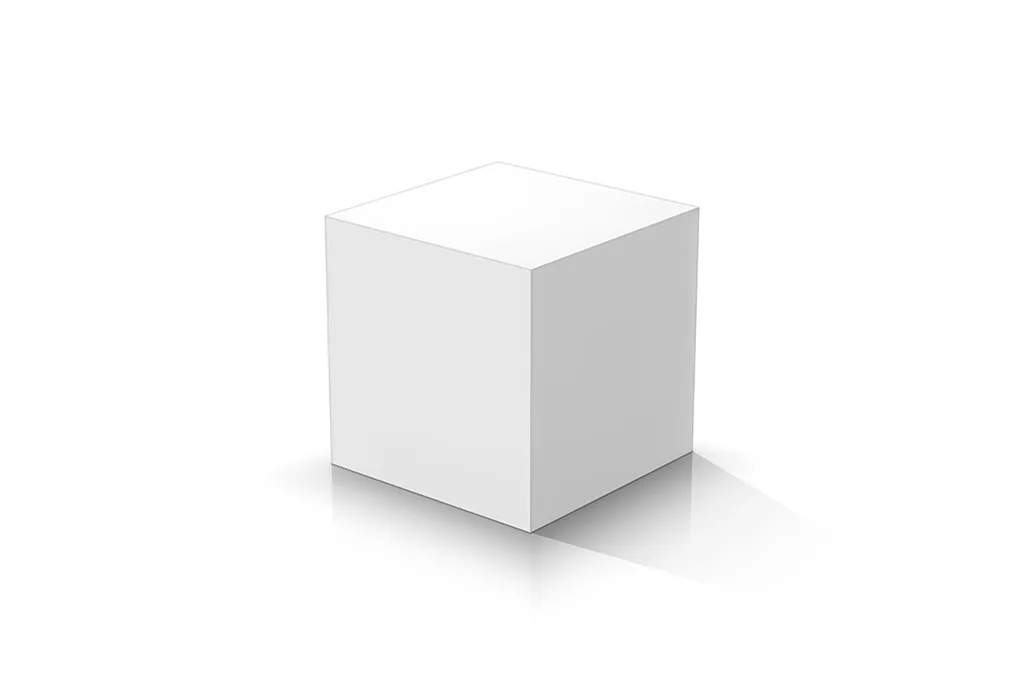
Properties of Dice:
1. It has 6 an identical sq. faces.
2. It has 12 equal edges.
3. All its angles are proper angles.
4. It has 8 vertices (corners).
2. Cuboid (Rectangular Prism)
Properties of Cuboid:
1. It has 6 faces, the place reverse faces are equal.
2. It has 12 edges.
3. All inside angles are proper angles.
4. It has 8 vertices.
3. Sphere
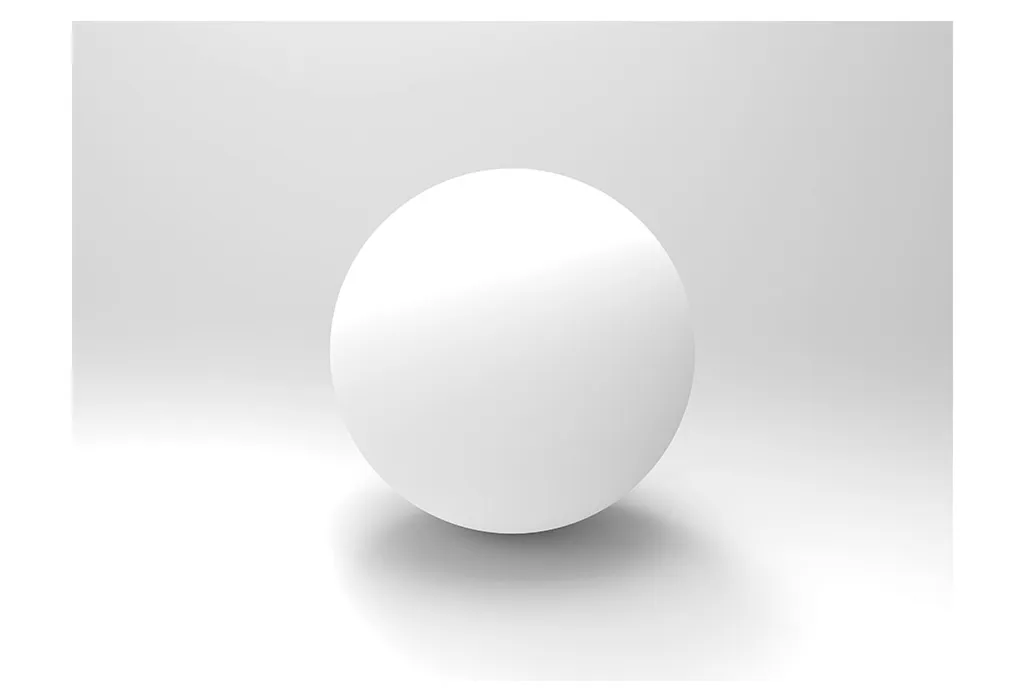
Properties of Sphere:
1. It has one steady curved floor.
2. It doesn't have any edges or vertices.
3. All factors on the floor are equidistant from its heart.
4. It has a continuing cross-section in any course.
4. Cylinder
Properties of Cylinder:
1. It has 2 flat, parallel circle bases and one curved floor.
2. It doesn't have any vertices.
3. The cross-section perpendicular to its base provides a circle.
4. The size between the 2 bases is named the peak.
5. Cone

Properties of Cone:
1. It has 1 flat round base and 1 curved floor.
2. It has a pointed vertex reverse its base.
3. The size from the bottom to the vertex is named the peak.
4. The straight line becoming a member of the vertex and the midpoint of the bottom circle is the axis.
6. Pyramid
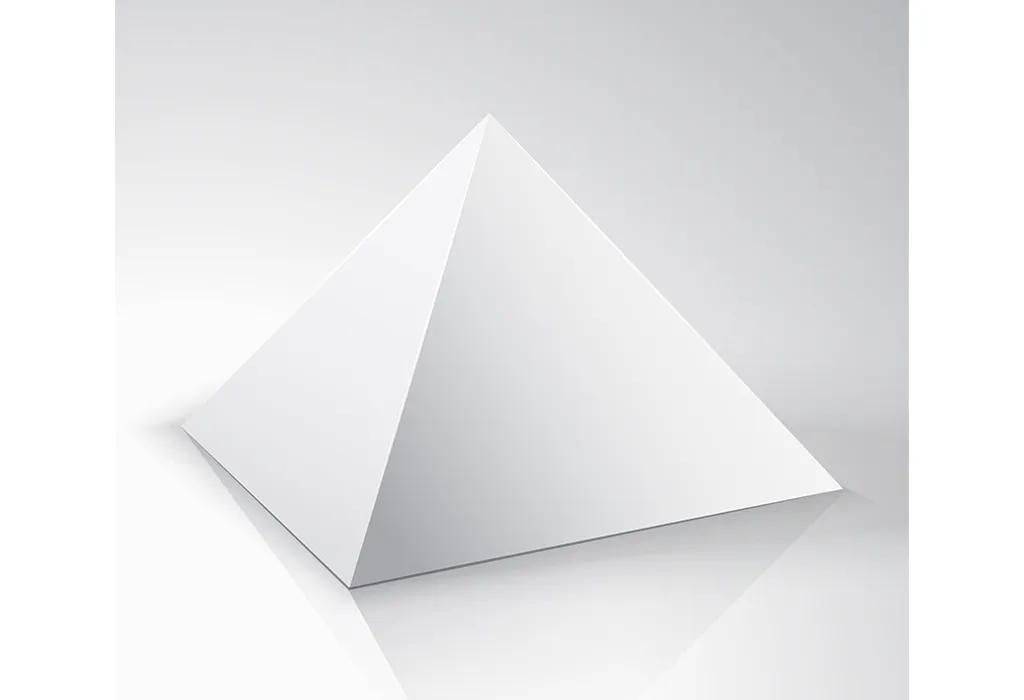
Properties of Pyramid:
1. It has a polygon base and triangular faces that converge to a single level referred to as the apex.
2. The variety of triangular faces equals the variety of sides within the base polygon.
3. The bottom may be any polygon, like a triangle, sq., or hexagon.
4. All of the triangular faces meet on the frequent vertex or apex.
7. Tetrahedron
Properties of Tetrahedron:
1. It's a pyramid with a triangular base.
2. It has 4 equilateral triangle faces.
3. It has 6 equal-length edges.
4. It has 4 vertices.
Understanding these properties might help in distinguishing between totally different stable shapes and supply a basis for extra complicated geometric rules.
What Are The Faces, Edges, And Vertices Of Strong Shapes?
Strong shapes are a major a part of geometry, with their properties and traits forming the premise of spatial understanding. An integral solution to distinguish and perceive stable shapes is by analyzing their faces, edges, and vertices. Every of those parts affords insights into the character, construction, and sort of the 3D object in query. Let’s delve deeper into every of those facets:
Faces
- A face is a flat or curved floor on a stable form. As an illustration, a dice has six flat faces, whereas a sphere has one curved face.
- The faces decide the form and sort of the 3D object. For instance, a pyramid’s faces are triangular, resulting in its distinctive construction.
Edges
- Edges are the road segments the place two faces of a stable form meet. A dice, for instance, has 12 edges.
- The quantity and size of edges might help in figuring out the stable form. A tetrahedron has 6 equal-length edges, distinguishing it from different pyramids.
Vertices
- A vertex (plural: vertices) is a degree the place two or extra edges meet. A dice has 8 vertices.
- The variety of vertices can typically point out the kind of stable form. As an illustration, a tetrahedron has 4 vertices, whereas an oblong prism or cuboid has 8.
Remembering these core parts and their traits is significant when exploring stable shapes for sophistication 1, 2, and three college students, making their journey on the planet of geometry easy and enlightening.
Vital Factors To Bear in mind About Strong Shapes
Strong shapes type the premise of our understanding of the three-dimensional world round us. Whether or not it’s the constructions we inhabit, the toys youngsters play with, or the pure types we see in nature, stable shapes are integral. Listed here are some essential factors to keep in mind when desirous about stable shapes:
1. Dimensionality: Not like 2D shapes which have solely size and width, stable shapes have a 3rd dimension – depth. That is what provides them quantity and makes them occupy house in the true world.
2. Uniformity will not be necessary: Strong shapes can have uniform faces and angles, like cubes, or they will have different faces and angles, like pyramids. Their selection is huge, spanning common shapes just like the tetrahedron to irregular ones like sure crystals.
3. Actual-world utility: Strong shapes are foundational in a number of disciplines reminiscent of structure, engineering, and design. Understanding their properties is important for these functions. As an illustration, the power of a cylindrical pillar versus a sq. one can decide architectural selections.
4. Floor and Quantity: Each stable form has each an exterior floor space and an inside quantity. The floor space is the overall space that the floor of the article occupies, whereas the quantity represents the house enclosed by the form. Each are important metrics in numerous real-world eventualities, from packaging design to development.
Remembering these key factors can present a stable basis (pun supposed!) for diving deeper into the world of geometry and appreciating the multifaceted nature of the 3D world round us.
Solved Examples Of Strong Shapes For Youngsters
Educating children about geometry generally is a enjoyable expertise, particularly when stable shapes are launched. Sensible, hands-on examples could make these ideas relatable and comprehensible. Listed here are some easy examples tailor-made for youngsters within the age group of 5-8 years:
Instance 1
Drawback:
Emma has a toy field within the form of a dice. All its sides are of the identical size. If one facet of the dice is 10 items lengthy, how lengthy is the alternative facet?
Answer:
For the reason that toy field is a dice, all its sides are of equal size. So, the alternative facet can be: = 10 items
Instance 2
Drawback:
Lucas is enjoying with a cube (which is a dice) and a toy pyramid. If the cube has 6 faces, what number of faces does the toy pyramid have?
Answer:
A pyramid has one base and triangular faces that meet on the prime. So, for a pyramid with a sq. base, it can have: = 1 base + 4 triangular faces = 5 faces
Instance 3
Drawback:
Liam has a toy within the form of a cylinder. He notices it has two flat faces and one curved face. What number of complete faces does the cylinder have?
Answer:
Complete variety of faces in a cylinder: = 2 flat faces + 1 curved face = 3 faces
Instance 4
Drawback:
Sophia has 3 marbles, every within the form of a sphere. If she seems to be at one marble, what number of flat faces can she discover?
Answer:
A sphere is a superbly spherical stable form. So, it has: = 0 flat faces
These hands-on, tangible stable shapes examples make it simpler for youngsters within the 5-8 years age group to visualise and perceive the essential properties of three-dimensional shapes in a enjoyable and fascinating method.
Apply Questions To Train Your Youngster About Strong Shapes
To check your little one’s understanding and make studying interactive, listed below are some apply questions associated to stable shapes for youths
Q1: Which stable form has just one flat face and one curved face?
Selections:
a) Dice
b) Cone
c) Sphere
d) Pyramid
Reply:
b) Cone
Q2: What number of vertices does a dice have?
Selections:
a) 6
b) 8
c) 4
d) 12
Reply:
b) 8
Q3: What form is a basketball most just like?
Selections:
a) Cylinder
b) Sphere
c) Dice
d) Prism
Reply:
b) Sphere
Bear in mind, whereas answering these questions, encourage your little one to visualise or draw the shapes if wanted. This not solely helps them perceive the properties of stable shapes higher but in addition boosts their spatial intelligence.
The exploration of “stable figures shapes” types an integral basis on the planet of geometry. From the homes we stay in to the toys youngsters play with, examples of stable shapes in actual life are throughout us, influencing our day-to-day experiences. This foundational data not solely deepens our understanding of the mathematical universe but in addition shapes our interplay with the bodily world. As youngsters start the method of naming stable shapes, they’re not simply studying summary ideas. As an alternative, they’re gaining instruments to categorize and perceive their environment. From recognizing a soccer ball as a sphere to figuring out a field of cereal as a cuboid, these recognitions act as bridges between theoretical data and tangible experiences. In essence, stable shapes present a lovely intersection of the summary and the true, fostering each studying and marvel.